COMPACTION MODEL

In sedimentary basins, the porosity of the sediment decreases in a regular fashion with depth as long as the pore fluid remains hydrostatic. The relationship between porosity and effective stress variations is derived in the following manner. We define a long-term porosity compressibility by:

, (1)

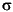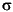where is the total interconnected porosity, _O is the porosity in an uncompacted reference state corresponding to the seafloor in sedimentary basins, _eff = - p, 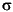is the total confining stress, which is the first invariant of the total stress tensor, and p is the total fluid pressure (equal to the hydrostatic fluid pressure, p_H, plus a fluid overpressure term, p). Palciauskas and Domenico (1989) show that compaction by pressure solution in sedimentary basins is irreversible and several orders of magnitude more effective than the corresponding poro-elastic (reversible) compressibility. They also show theoretically that does not depend on the effective stress itself. We can recast Equation 1 in the following form:

as , (2)

where will be considered as a constant as shown by Palciauskas and Domenico (1989). In the following, we will consider 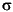_eff 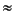(P - p) where P is the lithostatic stress. This approximation is valid only in absence of strong tectonic stresses, and with a Poisson's ratio close to 0.50 (Magara, 1978). The Poisson ratio for long-term deformation of mud or unconsolidated sediments such as observed at the sites investigated is close to 0.50 (Hamilton, 1971). The change in lithostatic and fluid pressures under hydrostatic conditions (subscript H) over a depth increment dz are given by (Magara, 1978):

, and (3)

 

, (4)

where _H is the "hydrostatic porosity" (Fig. 2), _f is the density of the fluid in the interconnected pore space, _m is the grain density, and g is acceleration of gravity. In the presence of gas, the fluid density _f can be related to the water saturation S_w, and to the gas density _g by _f = S_ww + (1-S_w)_g where _w is the saline water density. 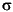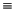Because, the differential of the effective stress is given by d_eff d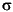 - dp = dP_H - dp_H, Equations 3 and 4 allow the compaction equation, Equation 2, to be rewritten:

. (5)

Integration from the seafloor (z = 0, = ₀) to depth z yields:

, (6)

where the characteristic depth z_c is defined by:

. (7)

Equation 6 can be rewritten to give:

. (8)

Equation 8 corresponds to the normal trend of compaction in sedimentary basins. Finally, the lithostatic pressure in a hydrostatically pressured section, P_H, can be obtained by substituting Equation 8 into Equation 3 and integrating from the surface to the depth z, yielding:

. (9)

Fluid overpressure is the result of a balance between the rate of overpressure generation and its dissipation by fluid flow (Palciauskas and Domenico, 1989). In this paper, we only consider fluid overpressures resulting from disequilibrium compaction. Disequilibrium compaction refers to the situation where sediments are unable to expel their pore fluids in response to sediment loading, which causes fluid overpressure. It is convenient to describe all parameters as departures from the values these parameters would have under hydrostatic conditions. Defining the departure from hydrostatic pore pressure, lithostatic pressure, and porosity by p, P, and , we can write the fluid pressure, the lithostatic pressure, and the porosity without loss of accuracy:

, (10)

, and (11)

, (12)

where p_H = _fgz is the hydrostatic fluid pressure, and P_H and _H are given by Equations 8 and 9, respectively. We call the "excess porosity" (Fig. 2). From Equations 2, 10, 11, and 12, the porosity change is related to the effective stress change, P - p, by:

. (13)

The lithostatic stress is related to the porosity by:

. (14)

Combining Equations 3, 11, 12, and 14, we have:

. (15)

Combining Equations 13 and 15, we see the excess fluid pressure and the excess porosity are related by:

, (16)

or alternatively, using the definition of the characteristic depth z_c, Equation 7,

. (17)

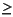The second term of Equation 17 represents the variation of the lithostatic pressure induced by variations of the excess porosity . Equations 13-17 are exact in the sense that they do not result from any approximation other than the constraint of disequilibrium compaction discussed above. Provided p 0, is necessarily positive.